ProductsAbaqus/StandardAbaqus/Explicit
Mises plasticity with isotropic hardening
Elements tested
C3D8
CPS4
T3D2
Problem description
Material:
- Elasticity
-
Young's modulus, E = 200.0E3
Poisson's ratio, = 0.3
- Plasticity
-
Hardening:
Yield stress Plastic strain 200. 0.0000 220. 0.0009 220. 0.0029
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
- mpliho3hut.inp
-
Uniaxial tension, C3D8 elements.
- mpliho2hut.inp
-
Uniaxial tension, CPS4 elements.
- mpliho1hut.inp
-
Uniaxial tension, T3D2 elements.
- mpliho3gsh.inp
-
Shear, C3D8 elements.
- mpliho2gsh.inp
-
Shear, CPS4 elements.
- mpliho1mcy.inp
-
Cyclic loading, T3D2 elements.
- mpliho3vlp.inp
-
Linear perturbation steps containing LOAD CASE, uniaxial tension, C3D8 elements.
- mplihi3hut.inp
-
Uniaxial tension with nonzero initial condition for , C3D8 elements.
![]()
Mises plasticity with linear kinematic hardening
Elements tested
T3D2
Problem description
Material:
- Elasticity
-
Young's modulus, E = 200.0E3
Poisson's ratio, = 0.3
- Plasticity
-
Hardening:
Yield stress Plastic strain 200. 0.0000 220. 0.0009
The linear kinematic hardening model is defined by the slope of the stress-strain data given earlier. (The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
Abaqus/Standard input files
- mplkho1mcy.inp
-
Cyclic loading, T3D2 elements.
- mplkhi1hut.inp
-
Uniaxial tension with nonzero initial condition for , load control, T3D2 elements.
Abaqus/Explicit input files
- mplkho1mcy_xpl.inp
-
Cyclic loading, T3D2 elements.
- mplkhi1hut_xpl.inp
-
Uniaxial tension with nonzero initial condition for , load control, T3D2 elements.
![]()
Mises plasticity with multilinear kinematic hardening
Elements tested
C3D8
Problem description
Material:
- Elasticity
-
Young's modulus, E = 200.0E3
Poisson's ratio, = 0.3
- Plasticity
-
Hardening:
Yield stress Plastic strain Temperature Field variable 2000. 0.00 0. 0. 3000. 0.25 0. 0. 5500. 1.0 0. 0. 800. 0. 100. 0. 2000. 0.25 100. 0. 4000. 0.8 100. 0. 400. 0. 0. 200. 800. 0.1 0. 200. 1600. 0.4 0. 200. 200. 0. 100. 200. 500. 0.5 100. 200. 750. 1. 100. 200. - Thermal properties
-
Coefficient of expansion, = 1.E-4
Results and discussion
The results agree well with the analytical solution.
Input files
Abaqus/Standard input file
- multilinkinhard_fv.inp
-
Uniaxial test, C3D8 element.
![]()
Mises plasticity with combined isotropic/kinematic hardening
Elements tested
B21
C3D8
C3D8R
CPE4
CPS4
M3D4
SAX1
T3D2
Problem description
Material 1
- Elasticity
-
Young's modulus, E = 200.0E3
Poisson's ratio, = 0.3
- Plasticity
-
Initial yield stress: = 200.0
Isotropic hardening parameter, = 2000
Isotropic hardening parameter, b = 0.25
Kinematic hardening parameter, C = 2.222 × 104
Kinematic hardening parameter, = 34.65
The parameters given above are used to generate data for some of the input files that use tabular data. (The units are not important.)
Material 2
- Elasticity
-
Young's modulus, E = 200.0E3
Poisson's ratio, = 0.3
- Plasticity
-
Initial yield stress: = 200.0
Kinematic hardening parameter, C = 2.222 × 104
Kinematic hardening parameter, = 0.0
The parameters given above are used to generate data for some of the input files that use tabular data. (The units are not important.)
Material 3
- Elasticity
-
Young's modulus, E = 200.0E3
Poisson's ratio, = 0.3
- Plasticity
-
Initial yield stress: = 200.0
Isotropic hardening parameter, = 0.0
Isotropic hardening parameter, b = 0.0
Kinematic hardening parameter, C = 2.222 × 104
Kinematic hardening parameter, = 34.65
(The units are not important.)
Material 4
- Elasticity
-
Young's modulus, E = 200.0E3
Poisson's ratio, = 0.3
- Plasticity
-
Initial yield stress: = 200.0
Isotropic hardening parameter, = 2000
Isotropic hardening parameter, b = 0.25
Kinematic hardening parameter, = 1.111 × 104
Kinematic hardening parameter, = 34.65
Kinematic hardening parameter, = 5.555 × 103
Kinematic hardening parameter, = 34.65
Kinematic hardening parameter, = 5.555 × 103
Kinematic hardening parameter, = 0.0
(The units are not important.)
Material 5
- Elasticity
-
Young's modulus, E = 200.0E3
Poisson's ratio, = 0.3
- Plasticity
-
Initial yield stress: = 200.0
Isotropic hardening parameter, = 0.0
Isotropic hardening parameter, b = 0.0
Kinematic hardening parameter, = 1.111 × 104
Kinematic hardening parameter, = 34.65
Kinematic hardening parameter, = 5.555 × 103
Kinematic hardening parameter, = 34.65
Kinematic hardening parameter, = 5.555 × 103
Kinematic hardening parameter, = 0.0
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
Abaqus/Standard input files
- mplchi3nt1mb.inp
-
Uniaxial tension, nonzero initial conditions for , , and ; displacement control; M3D4 elements with rebar; number backstresses = 3.
- mplchb2hut.inp
-
Uniaxial tension with temperature and field variable dependence, displacement control, SAX1 elements.
- mplchb3hut.inp
-
Uniaxial tension with temperature-dependent , displacement control, C3D8R elements.
- mplcho1hut.inp
-
Uniaxial tension, tabulated data, load control, B21 elements.
- mplcho1hutmb.inp
-
Uniaxial tension, tabulated data, load control, B21 elements, number backstresses = 3.
- mplcho3nt1.inp
-
Uniaxial tension, load control, C3D8 elements.
- mplchi3nt1.inp
-
Uniaxial tension, nonzero initial conditions for , , and ; displacement control; M3D4 elements with rebar.
- mplchi2hut.inp
-
Uniaxial tension with orientation and nonzero initial conditions for and , displacement control, CPE4 elements.
- mplcho1mcy.inp
-
Cyclic loading, no isotropic hardening, displacement control, T3D2 elements.
- mplcho1mcymb.inp
-
Cyclic loading, no isotropic hardening, displacement control, T3D2 elements, number backstresses = 3.
- mplcho2gsh.inp
-
Simple shear including perturbation step, CPS4 elements.
- mplchb2hutmb.inp
-
Uniaxial tension with temperature and field variable dependence, displacement control, SAX1 elements, number backstresses = 3.
- mplchi2hutmb.inp
-
Uniaxial tension with orientation and nonzero initial conditions for and , displacement control, CPE4 elements, number backstresses = 3.
- mplcho3nt1mb.inp
-
Uniaxial tension, load control, C3D8 elements, number backstresses = 3.
- mplcho2gshmb.inp
-
Simple shear including perturbation step, CPS4 elements, number backstresses = 3.
Abaqus/Explicit input files
- mplchi3nt1mb_xpl.inp
-
Uniaxial tension, nonzero initial conditions for , , and ; displacement control; M3D4R elements with rebar; number backstresses = 3.
- mplchb2hut_xpl.inp
-
Uniaxial tension with temperature and field variable dependence, displacement control, SAX1 elements.
- mplchb3hut_xpl.inp
-
Uniaxial tension with temperature-dependent , displacement control, C3D8R elements.
- mplcho1hut_xpl.inp
-
Uniaxial tension, tabulated data, load control, B21 elements.
- mplcho1hutmb_xpl.inp
-
Uniaxial tension, tabulated data, load control, B21 elements, number backstresses = 3.
- mplcho3nt1_xpl.inp
-
Uniaxial tension, load control, C3D8R elements.
- mplchi3nt1_xpl.inp
-
Uniaxial tension, nonzero initial conditions for , , and ; displacement control; M3D4R elements with rebar.
- mplchi2hut_xpl.inp
-
Uniaxial tension with orientation and nonzero initial conditions for and , displacement control, CPE4R elements.
- mplcho1mcy_xpl.inp
-
Cyclic loading, no isotropic hardening, displacement control, T3D2 elements.
- mplcho1mcymb_xpl.inp
-
Cyclic loading, no isotropic hardening, displacement control, T3D2 elements, number backstresses = 3.
- mplcho2gsh_xpl.inp
-
Simple shear including perturbation step, CPS4R elements.
- mplchb2hutmb_xpl.inp
-
Uniaxial tension with temperature and field variable dependence, displacement control, SAX1 elements, number backstresses = 3.
- mplchi2hutmb_xpl.inp
-
Uniaxial tension with orientation and nonzero initial conditions for and , displacement control, CPE4R elements, number backstresses = 3.
- mplcho3nt1mb_xpl.inp
-
Uniaxial tension, load control, C3D8R elements, number backstresses = 3.
- mplcho2gshmb_xpl.inp
-
Simple shear including perturbation step, CPS4R elements, number backstresses = 3.
![]()
Adiabatic Mises plasticity
Elements tested
C3D8
CPS4
T3D2
Problem description
Material:
- Elasticity
-
Young's modulus, E = 30.0E6
Poisson's ratio, = 0.3
- Plasticity
-
Hardening:
Yield stress Plastic strain Temperature 30.0E3 0.000 0.0 50.0E3 0.200 0.0 50.0E3 2.000 0.0 3.0E3 0.000 100.0 5.0E3 0.200 100.0 5.0E3 2.000 100.0 - Other properties
-
Density, = 1000.0
Specific heat, c = 0.4
Inelastic heat fraction = 0.5
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
- mhliho3hut.inp
-
Uniaxial tension, C3D8 elements.
- mhliho1hut.inp
-
Uniaxial tension, T3D2 elements.
- mhliho3gsh.inp
-
Shear, C3D8 elements.
- mhliho2gsh.inp
-
Shear, CPS4 elements.
- mhliho3ltr.inp
-
Triaxial, C3D8 elements.
- mhliht3hut.inp
-
Uniaxial tension, C3D8 elements.
- mhliht3xmx.inp
-
Multiaxial, C3D8 elements.
![]()
Hill plasticity
Elements tested
C3D8
Problem description
Material:
- Elasticity
-
Young's modulus, E = 200.0E3
Poisson's ratio, = 0.3
- Plasticity
-
Hardening:
Yield stress Plastic strain 200. 0.0000 220. 0.0009 220. 0.0029 Anisotropic yield ratios: 1.5, 1.2, 1.0, 1.0, 1.0, 1.0
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
- mppiho3nt1.inp
-
Uniaxial tension in direction 1, C3D8 elements.
- mppiho3ot2.inp
-
Uniaxial tension in direction 2, C3D8 elements.
- mppiho3pt3.inp
-
Uniaxial tension in direction 3, C3D8 elements.
- mppiho3vlp.inp
-
Linear perturbation steps containing LOAD CASE, uniaxial tension in direction 1, C3D8 elements.
![]()
Deformation plasticity
Elements tested
C3D8
CPS4
T3D2
Problem description
Material:
- Elasticity
-
Young's modulus, E = 200.0E3
Poisson's ratio, = 0.3
- Plasticity
-
Yield stress, = 200.0
Exponent, n = 21.315
Yield offset, = 0.11802
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
- mdfooo3hut.inp
-
Uniaxial tension, C3D8 elements.
- mdfooo3huti.inp
-
Uniaxial tension with initial stress, C3D8 elements.
- mdfooo2hut.inp
-
Uniaxial tension, CPS4 elements.
- mdfooo2huti.inp
-
Uniaxial tension with initial stress, CPS4 elements.
- mdfooo1hut.inp
-
Uniaxial tension, T3D2 elements.
- mdfooo1huti.inp
-
Uniaxial tension with initial stress, T3D2 elements.
![]()
Drucker-Prager plasticity with linear elasticity
Elements tested
C3D8
C3D8R
CAX4
CPE4
CPS4
Problem description
Material:
- Elasticity
-
Young's modulus, E = 300.0E3
Poisson's ratio, = 0.3
- Plasticity
-
Angle of friction, = 40.0
Dilation angle, = 40.0
Third invariant ratio, K = 0.78 (when included; otherwise, 1.0)
Hardening curve:
Yield stress Plastic strain 6.0E3 0.000000 9.0E3 0.020000 11.0E3 0.063333 12.0E3 0.110000 12.0E3 1.000000
(The units are not important.)
The hyperbolic and exponent forms of the yield criteria are verified by using parameters that reduce them into equivalent linear forms. Reducing the hyperbolic yield function into a linear form requires that . Reducing the exponent yield function into a linear form requires that b = 1.0 and that a = ()−1.
Results and discussion
Most tests in this section are set up as cases of the homogeneous deformation of a single element of unit dimensions. Consequently, the results are identical for all integration points within the element. To test certain conditions, however, it is necessary to set up inhomogeneous deformation problems. In each case the constitutive path is integrated with 20 increments of fixed size.
Input files
Shear criterion: linear Drucker-Prager
- mdeooo3euc.inp
-
Uniaxial compression, C3D8 elements.
- mdeooo2euc.inp
-
Uniaxial compression, CPS4 elements.
- mdeooo3ctc.inp
-
Triaxial compression, CAX4 elements.
- mdeooo3dte.inp
-
Triaxial extension, CAX4 elements.
- mdekoo3dte.inp
-
K = 0.78, triaxial extension, CAX4 elements.
- mdeooo3gsh.inp
-
Shear, C3D8 elements.
- mdeooo2gsh.inp
-
Shear, CPS4 elements.
- mdekoo3gsh.inp
-
K = 0.78, shear, C3D8 elements.
- mdekoo2gsh.inp
-
K = 0.78; shear, CPS4 elements.
- mdeooo3hut.inp
-
Uniaxial tension, C3D8 elements.
- mdeooo2hut.inp
-
Uniaxial tension, CPS4 elements.
- mdekoo3hut.inp
-
K = 0.78, uniaxial tension, C3D8 elements.
- mdekoo2hut.inp
-
K = 0.78, uniaxial tension, CPS4 elements.
- mdekot3hut.inp
-
K = 0.78, uniaxial tension with temperature dependence, C3D8R elements.
- mdeooo3jht.inp
-
Hydrostatic tension, C3D8 elements.
- mdeooo3ltr.inp
-
Triaxial stress, CPE4 elements (inhomogeneous).
- mdeooo2ltr.inp
-
Triaxial stress, CPS4 elements (inhomogeneous).
- mdekoo3ltr.inp
-
K = 0.78, triaxial stress, CPE4 elements (inhomogeneous).
- mdekoo2ltr.inp
-
K = 0.78, triaxial stress, CPS4 elements (inhomogeneous).
- mdeoot3euc.inp
-
Uniaxial compression with temperature dependence, C3D8 elements.
- mdeooo3vlp.inp
-
Linear perturbation uniaxial compression, C3D8 elements.
- mdedos3euc.inp
-
Uniaxial compression with rate dependence, C3D8 elements.
- mdeooi3euc.inp
-
Uniaxial compression with nonzero initial condition for , C3D8 elements.
- mdedoo2euc.inp
-
Uniaxial tension, perfect plasticity, CPS4 elements.
Shear criterion: exponent
- mdeeoo3jht.inp
-
Hydrostatic tension, C3D8 elements.
- mdeeoo3ltr.inp
-
Triaxial stress, CPE4 elements (inhomogeneous).
- mdeeoo3dte.inp
-
Triaxial extension, CAX4 elements.
- mdeeoo3hut.inp
-
Uniaxial tension, C3D8 elements.
- mdeeoo2hut.inp
-
Uniaxial tension, CPS4 elements.
- mdeeoo3gsh.inp
-
Shear, C3D8 elements.
- mdeeoo2gsh.inp
-
Shear, CPS4 elements.
- mdeeoo3ctc.inp
-
Triaxial compression, CAX4 elements.
- mdeeoo3euc.inp
-
Uniaxial compression, C3D8 elements.
- mdeeoo2euc.inp
-
Uniaxial compression, CPS4 elements.
- mdeeos3euc.inp
-
Uniaxial compression with rate dependence, C3D8 elements.
- mdeeot3euc.inp
-
Uniaxial compression with temperature dependence, C3D8 elements.
- mdeeoo3vlp.inp
-
Linear perturbation uniaxial compression, C3D8 elements.
- mdeeoo3jht_xpl.inp
-
Hydrostatic tension, C3D8R elements.
- mdeeoo3ltr_xpl.inp
-
Triaxial stress, CPE4R elements (inhomogeneous).
- mdeeoo3dte_xpl.inp
-
Triaxial extension, CAX4R elements.
- mdeeoo3hut_xpl.inp
-
Uniaxial tension, C3D8R elements.
- mdeeoo2hut_xpl.inp
-
Uniaxial tension, CPS4R elements.
- mdeeoo3gsh_xpl.inp
-
Shear, C3D8R elements.
- mdeeoo2gsh_xpl.inp
-
Shear, CPS4R elements.
- mdeeoo3ctc_xpl.inp
-
Triaxial compression, CAX4R elements.
- mdeeoo3euc_xpl.inp
-
Uniaxial compression, C3D8R elements.
- mdeeoo2euc_xpl.inp
-
Uniaxial compression, CPS4R elements.
- mdeeos3euc_xpl.inp
-
Uniaxial compression with rate dependence, C3D8R elements.
- mdeeot3euc_xpl.inp
-
Uniaxial compression with temperature dependence, C3D8R elements.
Shear criterion: exponent with test data
- mdeeto3jht.inp
-
Hydrostatic tension, C3D8 elements.
- mdeeto3ltr.inp
-
Triaxial stress, CPE4 elements (inhomogeneous).
- mdeeto3dte.inp
-
Triaxial extension, CAX4 elements.
- mdeeto3hut.inp
-
Uniaxial tension, C3D8 elements.
- mdeeto2hut.inp
-
Uniaxial tension, CPS4 elements.
- mdeeto3gsh.inp
-
Shear, C3D8 elements.
- mdeeto2gsh.inp
-
Shear, CPS4 elements.
- mdeeto3ctc.inp
-
Triaxial compression, CAX4 elements.
- mdeeto3euc.inp
-
Uniaxial compression, C3D8 elements.
- mdeeto2euc.inp
-
Uniaxial compression, CPS4 elements.
- mdeets3euc.inp
-
Uniaxial compression with rate dependence, C3D8 elements.
- mdeeto3vlp.inp
-
Linear perturbation uniaxial compression, C3D8 elements.
- mdeeto3jht_xpl.inp
-
Hydrostatic tension, C3D8R elements.
- mdeeto3ltr_xpl.inp
-
Triaxial stress, CPE4R elements (inhomogeneous).
- mdeeto3dte_xpl.inp
-
Triaxial extension, CAX4R elements.
- mdeeto3hut_xpl.inp
-
Uniaxial tension, C3D8R elements.
- mdeeto2hut_xpl.inp
-
Uniaxial tension, CPS4R elements.
- mdeeto3gsh_xpl.inp
-
Shear, C3D8R elements.
- mdeeto2gsh_xpl.inp
-
Shear, CPS4R elements.
- mdeeto3ctc_xpl.inp
-
Triaxial compression, CAX4R elements.
- mdeeto3euc_xpl.inp
-
Uniaxial compression, C3D8R elements.
- mdeeto2euc_xpl.inp
-
Uniaxial compression, CPS4R elements.
- mdeets3euc_xpl.inp
-
Uniaxial compression with rate dependence, C3D8R elements.
Shear criterion: hyperbolic
- mdehoo3jht.inp
-
Hydrostatic tension, C3D8 elements.
- mdehoo3ltr.inp
-
Triaxial stress, CPE4 elements (inhomogeneous).
- mdehoo3dte.inp
-
Triaxial extension, CAX4 elements.
- mdehoo3hut.inp
-
Uniaxial tension, C3D8 elements.
- mdehoo2hut.inp
-
Uniaxial tension, CPS4 elements.
- mdehoo3gsh.inp
-
Shear, C3D8 elements.
- mdehoo2gsh.inp
-
Shear, CPS4 elements.
- mdehoo3ctc.inp
-
Triaxial compression, CAX4 elements.
- mdehoo3euc.inp
-
Uniaxial compression, C3D8 elements.
- mdehoo2euc.inp
-
Uniaxial compression, CPS4 elements.
- mdehos3euc.inp
-
Uniaxial compression with rate dependence, C3D8 elements.
- mdehot3euc.inp
-
Uniaxial compression with temperature dependence, C3D8 elements.
- mdehoo3vlp.inp
-
Linear perturbation uniaxial compression, C3D8 elements.
- mdehoo3jht_xpl.inp
-
Hydrostatic tension, C3D8R elements.
- mdehoo3ltr_xpl.inp
-
Triaxial stress, CPE4R elements (inhomogeneous).
- mdehoo3dte_xpl.inp
-
Triaxial extension, CAX4R elements.
- mdehoo3hut_xpl.inp
-
Uniaxial tension, C3D8R elements.
- mdehoo2hut_xpl.inp
-
Uniaxial tension, CPS4R elements.
- mdehoo3gsh_xpl.inp
-
Shear, C3D8R elements.
- mdehoo2gsh_xpl.inp
-
Shear, CPS4R elements.
- mdehoo3ctc_xpl.inp
-
Triaxial compression, CAX4R elements.
- mdehoo3euc_xpl.inp
-
Uniaxial compression, C3D8R elements.
- mdehoo2euc_xpl.inp
-
Uniaxial compression, CPS4R elements.
- mdehos3euc_xpl.inp
-
Uniaxial compression with rate dependence, C3D8R elements.
- mdehot3euc_xpl.inp
-
Uniaxial compression with temperature dependence, C3D8R elements.
Transferring results between Abaqus/Standard and Abaqus/Explicit
- sx_s_druckerprager.inp
-
Base problem for carrying out import from Abaqus/Standard to Abaqus/Explicit, C3D8R elements, uniaxial tension.
- sx_x_druckerprager_y_y.inp
-
Explicit dynamic continuation of sx_s_druckerprager.inp with both the reference configuration and the state imported, C3D8R elements, uniaxial tension.
- sx_x_druckerprager_n_y.inp
-
Explicit dynamic continuation of sx_s_druckerprager.inp with only the state imported, C3D8R elements, uniaxial tension.
- sx_x_druckerprager_n_n.inp
-
Explicit dynamic continuation of sx_s_druckerprager.inp without importing the state or the reference configuration, C3D8R elements, uniaxial tension.
- xs_s_druckerprager_y_y.inp
-
Import into Abaqus/Standard from sx_x_druckerprager_y_y.inp with both the reference configuration and the state imported, C3D8R elements, uniaxial tension.
- xs_s_druckerprager_n_y.inp
-
Import into Abaqus/Standard from sx_x_druckerprager_n_y.inp with only the state imported, C3D8R elements, uniaxial tension.
- xs_s_druckerprager_n_n.inp
-
Import into Abaqus/Standard from sx_x_druckerprager_n_n.inp without importing the state or the reference configuration, C3D8R elements, uniaxial tension.
![]()
Drucker-Prager plasticity with porous elasticity
Elements tested
CAX4
Problem description
Material:
- Elasticity
-
Logarithmic bulk modulus, = 1.49
Poisson's ratio, = 0.1
- Plasticity
-
Angle of friction, = 10.0
Dilation angle, = 10.0
Hardening curve:
Yield stress Plastic strain 100.0 0.0 500.0 0.5 - Initial conditions
-
Initial void ratio, = 4.1
The hyperbolic and exponent forms of the yield criteria are verified by using parameters that reduce them into equivalent linear forms. Reducing the hyperbolic yield function into a linear form requires that . Reducing the exponent yield function into a linear form requires that b = 1.0 and that a = ()−1.
(The units are not important.)
Results and discussion
The tests in this section are set up as cases of homogeneous deformation of a single element of unit dimensions. Consequently, the results are identical for all integration points within the element. In each case the constitutive path is integrated with 20 increments of fixed size.
Input files
Shear criterion: linear Drucker-Prager
- mdpdoo3bus.inp
-
Uniaxial strain, CAX4 elements.
- mdpdoo3ctc.inp
-
Triaxial compression, CAX4 elements.
Shear criterion: exponent
- mdpeoo3bus.inp
-
Uniaxial strain, CAX4 elements.
- mdpeoo3ctc.inp
-
Triaxial compression, CAX4 elements.
Shear criterion: exponent with test data
- mdpeto3bus.inp
-
Uniaxial strain, CAX4 elements.
- mdpeto3ctc.inp
-
Triaxial compression, CAX4 elements.
Shear criterion: hyperbolic
- mdphoo3bus.inp
-
Uniaxial strain, CAX4 elements.
- mdphoo3ctc.inp
-
Triaxial compression, CAX4 elements.
![]()
Cap plasticity
Elements tested
C3D8R
CAX4
CPE4
Problem description
Material:
In the tests described in this section, the following data for linear elasticity, cap plasticity I, cap hardening I, and K = 1.0 are used unless otherwise specified. With this data, the elastic shear modulus is 5000.0 and the bulk modulus is 10000.0. First yield in pure shear occurs at S12 = 100.0, first yield in pure hydrostatic compression occurs at PRESS = 270.0, first yield in pure hydrostatic tension occurs at PRESS = 300.0, and first yield with PRESS = occurs at PRESS = 120.0 and S12 = 125.0. C3D8 elements are used unless otherwise specified.
- Linear elasticity (used in nearly all tests)
-
Young's modulus, E = 12857.1429
Poisson's ratio, = 0.28571429 (= 1/7)
- Cap plasticity I (used in nearly all tests)
-
Cohesion, d = 173.20508 (= 100)
Slope of Drucker-Prager failure surface, = 30.0
Cap ellipticity, R = 0.61858957
Initial volumetric plastic strain, = 0.027
Transition parameter, = 0.69258232
Third invariant factor, K = 1.0 or 0.8, depending on the test.
- Cap hardening I (used in nearly all tests)
-
Position of the yield surface in pure hydrostatic compression,
Volumetric compressive plastic strain,
213.0 0.00 222.0 0.01 242.0 0.02 282.0 0.03 362.0 0.04 522.0 0.05 842.0 0.06 1482.0 0.07 2762.0 0.08 - Cap plasticity II
-
d = 0.2286E6
= 85.0
R = 0.0875
= 1.22
= 0.07877
K = 1.0
- Cap hardening II
-
Position of the yield surface in pure hydrostatic compression,
Volumetric compressive plastic strain,
0.03E6 0.0 0.20E6 1.22 2.00E6 2.44 2.00E7 3.66 - Porous elasticity I
-
Logarithmic bulk modulus, = 20.0
Poisson's ratio, = 0.28571429
Tensile strength limit, = 1.0E5
- Porous elasticity II
-
= 0.09
= 0.0
= 0.02E6
- Initial conditions
-
Initial void ratio, = 1.0
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
- mcaooo3mcy.inp
-
Hydrostatic cyclic test, displacement control.
The following six steps are executed:
-
Load, yielding in hydrostatic compression
-
Unload, still in compression
-
Reload, yielding in compression
-
Unload in compression and load, yielding in tension
-
Unload in tension and load, yielding in compression
-
Unload
-
- mcaooo3euc.inp
-
Uniaxial compressive stress test; displacement control.
Step 2 reverses the displacement causing yielding in tension.
- mcaooo3gsh.inp
-
Shear test; load control (S22 = −S11); overlaid soft linear element.
There will be some hydrostatic stress due to transverse restraint.
- mcaooo3ucs.inp
-
Cyclical shear test; displacement control; S12 dominant.
- mcaoot3ctc.inp
-
Hydrostatic compression to , then pure shear; displacement control; temperature dependence.
Yielding should be volume preserving.
- mcakoo3gsh.inp
-
Shear test; load control; two primary elements and two overlaid soft elements.
One set loaded with principal stresses (1, 1, −2), the other with (−1, −1, 2).
The ratio of yield stresses should be K = 0.8.
- mcaoob3bus.inp
-
Uniaxial compressive strain (odometer) test; CPE4 element; load control; with temperature and field variable dependence of the CAP PLASTICITY and CAP HARDENING data.
The temperatures and field variables are specified to give CAP PLASTICITY and CAP HARDENING data exactly the same as cap plasticity I and cap hardening I data.
- mcaooo3bus.inp
-
Uniaxial compressive strain (odometer) test; load control; NLGEOM and porous elasticity I.
The tangent bulk and shear moduli of porous elasticity I differ from that of the linear elasticity by about 1% over the strain range of the test.
- mcaooo3ctc.inp
-
Triaxial test. Hydrostatic loading to , then increase S11 only.
- mcaooo3vlp.inp
-
Uniaxial compressive strain (odometer) test; load control; the nonlinear analysis is split into two steps, each of which is preceded by a linear perturbation step.
The results of the nonlinear steps should correspond to those of mca0003bus.inp.
The results of the two linear perturbation steps (STATIC) should be identical because small displacements are assumed and the elasticity is linear.
- mcakoo3ltr.inp
-
A displacement pattern designed to produce different stress states at the 8 Gauss points but dominated by shear.
The aim is to test the robustness of the Newton loops, so very large strain increments are taken.
Displacement control. K = 0.8.
- mcaooo3ltr.inp
-
Another test of the robustness of the algorithm.
CAX4 element, porous elasticity II, cap plasticity II, and cap hardening II is used.
- mcaooo3xmx.inp
-
Tests adjustment of the initial position of the cap.
Two C3D8R elements with different initial stress states.
The initial stress in element 1 will cause an adjustment that will make the stress point lie on the cap yield surface.
The initial stress in element 2 will cause an adjustment that will make the stress point lie on the transition yield surface.
![]()
Clay plasticity with porous elasticity
Elements tested
C3D8
CAX8R
Problem description
Material:
- Porous elasticity
-
Logarithmic bulk modulus, = 0.026
Poisson's ratio, = 0.3
- Plasticity
-
Logarithmic plastic bulk modulus, = 0.174
Critical state slope, M = 1.0
Initial yield surface size, = 58.3
(except in tests mclxxxxahc.inp where we use = 130.9 or = 1.904)
Cap parameter, = 0.5 (when included; otherwise, 1.0)
Third invariant ratio, K = 0.78 (when included; otherwise, 1.0)
- Initial conditions
-
Initial void ratio, = 1.08
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
- mclooo3ahc.inp
-
Hydrostatic compression, C3D8 elements.
- mcloio3ahc.inp
-
Hydrostatic compression with intercept option, C3D8 elements.
- mclooo3ctc.inp
-
Triaxial compression, CAX8R elements.
- mclott3ctc.inp
-
Triaxial compression, temperature dependence, CAX8R elements.
- mclobo3ctc.inp
-
= 0.5, triaxial compression, CAX8R elements.
- mclooo3dte.inp
-
Triaxial extension, CAX8R elements.
- mclkoo3dte.inp
-
K = 0.78, triaxial extension, CAX8R elements.
- mclktd3dte.inp
-
K = 0.78, triaxial extension, field variable dependence, CAX8R elements.
- mclkbo3dte.inp
-
= 0.5, K = 0.78, triaxial extension, CAX8R elements.
- mcloto3euc.inp
-
Uniaxial compression, CAX8R elements.
- mclooo3gsh.inp
-
Shear, C3D8 elements.
- mcloto3gsh.inp
-
Shear, tabulated hardening, C3D8 elements.
- mclooo3vlp.inp
-
Linear perturbation hydrostatic compression, C3D8 elements.
![]()
Crushable foam plasticity
Elements tested
C3D8
CPE4
Problem description
Material:
- Elasticity
-
Young's modulus, E = 3.0E6
Poisson's ratio, = 0.2
- Plasticity
-
Initial yield stress in hydrostatic compression, = 2.0E5
Strength in hydrostatic tension, = 2.0E4
Initial yield stress in uniaxial compression, = 2.2E5
Yield stress ratio, = 1.1
Yield stress ratio, = 0.1
Hardening curve (from uniaxial compression):
Yield stress Plastic strain 2.200E5 0.0 2.465E5 0.1 2.729E5 0.2 2.990E5 0.3 3.245E5 0.4 3.493E5 0.5 3.733E5 0.6 3.962E5 0.7 4.180E5 0.8 4.387E5 0.9 4.583E5 1.0 4.938E5 1.2 5.248E5 1.4 5.515E5 1.6 5.743E5 1.8 5.936E5 2.0 6.294E5 2.5 6.520E5 3.0 6.833E5 5.0 6.883E5 10.0 - Initial conditions
-
Initial volumetric compacting plastic strain, , is set to 0.02 for the cases in which specifying an initial equivalent plastic strain is tested.
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
- mfeoto3ahc.inp
-
Hydrostatic compression, C3D8 elements.
- mfeoto3euc.inp
-
Uniaxial compression, C3D8 elements.
- mfeoto3gsh.inp
-
Shear, C3D8 elements.
- mfeoto3hut.inp
-
Uniaxial tension, C3D8 elements.
- mfeoti3euc.inp
-
Uniaxial compression, CPE4 elements.
- mfeoto3ltr.inp
-
Triaxial stress, CPE4 elements (inhomogeneous).
- mfeoto3vlp.inp
-
Linear perturbation with LOAD CASE and hydrostatic compression, C3D8 elements.
![]()
Clay plasticity with linear elasticity
Elements tested
C3D8
C3D8R
CAX4R
CAX8R
CPE4R
Problem description
Material 1
- Elasticity
-
The Young's modulus used in each test is given in the input file description. The modulus of each test is based on the average elastic stiffness of the equivalent test with porous elasticity at increments 10 and 20. A direct comparison with the results documented in Drucker-Prager plasticity with linear elasticity is, therefore, possible.
Poisson's ratio, = 0.3
- Plasticity
-
Critical state slope, M = 1.0
Initial volumetric plastic strain, = 0.4
Cap parameter, = 0.5 (when included; otherwise, 1.0)
Third invariant ratio, K = 0.78 (when included; otherwise, 1.0)
The exponential hardening curve used in Drucker-Prager plasticity with linear elasticity is entered in tabulated form with an initial volumetric plastic strain that corresponds to a yield surface size of either = 58.3 or = 130.9.
(The units are not important.)
Material 2
- Elasticity
-
Young's modulus, = 18820
Poisson's ratio, = 0.3
- Plasticity
-
Critical state slope, M = 1.0
Initial volumetric plastic strain, = 0.0
Cap parameter, = 1.0
Third invariant ratio, K = 1.0
Tabulated curves are used for defining the compressive and tensile hardening.
- Softening regularization
-
= 0.8
= 2.0
= 2.5
(The units are not important.)
Material 3
- Elasticity
-
Young's modulus, = 18820
Poisson's ratio, = 0.3
- Plasticity
-
Critical state slope, M = 1.0
Initial volumetric plastic strain, = 0.0
Cap parameter, = 1.0
Third invariant ratio, K = 1.0
Tabulated curves are used for defining the compressive and tensile hardening.
- Softening regularization
-
= 0.5
= 1.0
= 2.5
(The units are not important.)
Material 4 [Crook et al. (2002)]
- Elasticity
-
Engineering constants 200000.0 342000.0 342000.0 0.32 0.32 0.32 89900.0 89900.0 129545.5 - Plasticity
-
Critical state slope, M = 1.0
Initial volumetric plastic strain, = 0.0
Cap parameter, = 1.0
Third invariant ratio, K = 1.0
Tabulated curves are used for defining the compressive and tensile hardening.
Anisotropic yield ratios: 1.2, 1.0, 1.0, 0.71, 0.71, 0.99
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
Abaqus/Standard input files
Material 1:- mceooo3ahc.inp
-
Hydrostatic compression, C3D8 elements, E = 18820.
- mceoot3ctc.inp
-
Triaxial compression, CAX8R elements, E = 30732.
- mceobo3ctc.inp
-
= 0.5, triaxial compression, CAX8R elements, E = 29556.
- mceooo3dte.inp
-
Triaxial extension, CAX8R elements, E = 21114.
- mcekod3dte.inp
-
K = 0.78, triaxial extension, CAX8R elements, E = 28140.
- mcekbo3dte.inp
-
= 0.5, K = 0.78, triaxial extension, CAX8R elements, E = 27580.
- mceooo3euc.inp
-
Uniaxial compression, CAX8R elements, E = 30000.
- mceooo3gsh.inp
-
Shear, C3D8 elements, E = 2798.
- mceooo3vlp.inp
-
Linear perturbation with LOAD CASE and hydrostatic compression, C3D8 elements, E = 18820.
- clayteninipssoft3d.inp
-
Hydrostatic compression, C3D8 and C3D8R elements.
- clayteninipssoftgpe.inp
-
Hydrostatic compression, CAX4R elements.
- clayorthelaspoten3d.inp
-
Uniaxial compression and shear, C3D8R elements.
- clayorthelaspotengpe.inp
-
Shear, CPE4R elements.
Abaqus/Explicit input files
Material 1:- mceooo3ahc_xpl.inp
-
Hydrostatic compression, C3D8 elements, E = 18820.
- mceoot3ctc_xpl.inp
-
Triaxial compression, CAX4R elements, E = 30732.
- mceobo3ctc_xpl.inp
-
= 0.5, triaxial compression, CAX4R elements, E = 29556.
- mceooo3dte_xpl.inp
-
Triaxial extension, CAX4R elements, E = 21114.
- mcekod3dte_xpl.inp
-
K = 0.78, triaxial extension, CAX4R elements, E = 28140.
- mcekbo3dte_xpl.inp
-
= 0.5, K = 0.78, triaxial extension, CAX4R elements, E = 27580.
- mceooo3euc_xpl.inp
-
Uniaxial compression, CAX4R elements, E = 30000.
- mceooo3gsh_xpl.inp
-
Shear, C3D8 elements, E = 2798.
- clayteninipssoft3d_xpl.inp
-
Hydrostatic compression, C3D8 and C3D8R elements.
- clayteninipssoftgpe_xpl.inp
-
Hydrostatic compression, CAX4R elements.
- clayorthelaspoten3d_xpl.inp
-
Uniaxial compression and shear, C3D8R elements.
- clayorthelaspotengpe_xpl.inp
-
Shear, CPE4R elements.
Transferring results from Abaqus/Standard to Abaqus/Explicit
Material 1:- mceooo3ahc_sx_s.inp
-
Abaqus/Standard analysis, hydrostatic compression, C3D8 elements, E = 18820.
- mceooo3ahc_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mceoot3ctc_sx_s.inp
-
Abaqus/Standard analysis, triaxial compression, CAX4R elements, E =27580.
- mceoot3ctc_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mceobo3ctc_sx_s.inp
-
Abaqus/Standard analysis, = 0.5, triaxial compression, CAX4R elements, E =29556.
- mceobo3ctc_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mceooo3dte_sx_s.inp
-
Abaqus/Standard analysis, triaxial extension, CAX4R elements, E =21114.
- mceooo3dte_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mcekod3dte_sx_s.inp
-
Abaqus/Standard analysis, K = 0.78, triaxial extension, CAX4R elements, E =28140.
- mcekod3dte_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mcekbo3dte_sx_s.inp
-
Abaqus/Standard analysis, = 0.5, K = 0.78, triaxial extension, CAX4R elements, E =27580.
- mcekbo3dte_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mceooo3euc_sx_s.inp
-
Abaqus/Standard analysis, uniaxial compression, CAX4R elements, E =30000.
- mceooo3euc_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mceooo3gsh_sx_s.inp
-
Abaqus/Standard analysis, shear, C3D8 elements, E =2798.
- mceooo3gsh_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
Transferring results from Abaqus/Explicit to Abaqus/Standard
Material 1:- mceoot3ctc_xs_x.inp
-
Abaqus/Explicit analysis, triaxial compression, CAX4R elements, E =27580.
- mceoot3ctc_xs_s.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
- mceooo3dte_xs_x.inp
-
Abaqus/Explicit analysis, triaxial extension, CAX4R elements, E =21114.
- mceooo3dte_xs_s.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
- mceooo3gsh_xs_x.inp
-
Abaqus/Explicit analysis, shear, C3D8 elements, E =2798.
- mceooo3gsh_xs_s.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
- mceobo3ctc_xs_x.inp
-
Abaqus/Explicit analysis, = 0.5, triaxial compression, CAX4R elements, E =29556.
- mceobo3ctc_xs_s.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
- mcekod3dte_xs_x.inp
-
Abaqus/Explicit analysis, K = 0.78, triaxial extension, CAX4R elements, E =28140.
- mcekod3dte_xs_s.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
- mceooo3ahc_xs_x.inp
-
Abaqus/Explicit analysis, hydrostatic compression, C3D8 elements, E = 18820.
- mceooo3ahc_xs_s.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
References
- “Development of an Orthotropic 3D Elastoplastic Material Model for Shale,” SPE/ISRM Paper SPE 78238, 2002.
![]()
Porous metal plasticity
Elements tested
C3D8
CAX4
CAX4T
CPE4
Problem description
Material:
- Elasticity
-
Young's modulus, E = 300.0
Poisson's ratio, = 0.3
- Plasticity
-
Hardening curve:
Yield stress Plastic strain 1.0000000 0.00 1.7411011 0.05 2.7276924 0.50 2.9950454 0.80 - Porous metal plasticity
-
Modified Gurson's model: = 1.5, = 1.0, = 2.25
(otherwise, = = = 1.0)
Void nucleation parameters (when included): = 0.3, = 0.1, = 0.04
Initial relative density, = 0.95 ( = 0.05).
Material properties used in coupled temperature-displacement analysis
- Elasticity
-
Young's modulus, E = 200.0E9
Poisson's ratio, = 0.3
- Plasticity
-
Hardening curve:
Yield stress Plastic strain 7.0E8 0.00 3.7E9 10.0 - Porous metal plasticity
-
= = = 1.0
Initial relative density, = 0.95 ( = 0.05).
- Thermal properties
-
Specific heat, = 586.0
Density, = 7833.0
Conductivity, k = 52.0
Coefficient of expansion, = 1.2E−5
General:
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
- mgrono2xmx.inp
-
Inhomogeneous deformation, displacement control, CPE4 elements.
- mgrono2xmx1.inp
-
Same as mgrono2xmx.inp except that the initial relative density is specified using the INITIAL CONDITIONS, TYPE = RELATIVE DENSITY option.
- mgrono3hut.inp
-
Uniaxial tension, traction control, nucleation of voids, C3D8 elements.
- mgrono3jht.inp
-
Hydrostatic tension, displacement control, nucleation of voids, C3D8 elements.
- mgrooo2bus.inp
-
Uniaxial strain (confined compression), traction control, CAX4 elements.
- mgrooo2euc.inp
-
Uniaxial compression, traction control, CAX4 elements.
- mgrooo2gsh.inp
-
Shear, CPE4 elements.
- mgrooo2hut.inp
-
Uniaxial tension, displacement control, CAX4 elements.
- mgrooo2jht.inp
-
Hydrostatic tension, displacement control, CAX4 elements.
- mgrooo3gsh.inp
-
Shear, C3D8 elements.
- mgrooo3jht.inp
-
Hydrostatic tension, displacement control, C3D8 elements.
- mgrqno2hut.inp
-
Modified Gurson's model, uniaxial tension, displacement control, nucleation of voids, CAX4 elements.
- mgrqoo2ahc.inp
-
Modified Gurson's model, hydrostatic compression, displacement control, CAX4 elements.
- mgtooo2hut.inp
-
Uniaxial tension, coupled temperature-displacement, CAX4T elements.
- mgroob2hut.inp
-
Uniaxial tension, displacement control, CAX4 elements, temperature and field variable dependencies.
- mgrqnt2hut.inp
-
Modified Gurson's model, uniaxial tension, nucleation of voids, temperature dependencies.
![]()
Mohr-Coulomb plasticity
Elements tested
C3D8
C3D8R
CAX4
CAX4R
CPE4
CPE4R
Problem description
Material 1
- Elasticity
-
Young's modulus, E = 300.E3
Poisson's ratio, = 0.3
- Plasticity
-
Angle of friction, = 40°
Dilation angle, = 40°
Cohesion hardening curve:
Yield stress Plastic strain 6.0E3 0.000000 9.0E3 0.020000 11.0E3 0.063333 12.0E3 0.110000 12.0E3 1.000000 - Tension cutoff
-
Perfectly plastic, yield stress = 600.0
(The units are not important.)
Material 2
- Elasticity
-
Young's modulus, E = 300.E3
Poisson's ratio, = 0.3
- Plasticity
-
Angle of friction, = 30°
Dilation angle, = 20°
Cohesion hardening curve:
Yield stress Plastic strain 866.025 0.0 1732.05 1.0 - Tension cutoff
-
Softening response:
Yield stress Plastic strain 1000.0 0.0 100.0 1.0
(The units are not important.)
Material 3
- Elasticity
-
Young's modulus, E = 2 .E7
Poisson's ratio, = 0.3
- Plasticity
-
Angle of friction, = 30°
Dilation angle, = 20°
Perfectly plastic cohesion:
Yield stress Plastic strain 1000.0 0.0 1000.0 1.0 - Tension cutoff
-
Perfectly plastic:
Yield stress Plastic strain 1000.0 0.0 1000.0 1.0
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
Abaqus/Standard input files
Material 1:- mmoooo3jht.inp
-
Hydrostatic tension, C3D8 elements.
- mmoooo3ltr.inp
-
Triaxial stress, CPE4 elements (inhomogeneous).
- mmoooo3dte.inp
-
Triaxial extension, CAX4 elements.
- mmoooo3hut.inp
-
Uniaxial tension, C3D8 elements.
- mmoooo3gsh.inp
-
Shear, C3D8 elements.
- mmoooo3ctc.inp
-
Triaxial compression, CAX4 elements.
- mmoooo3euc.inp
-
Uniaxial compression, C3D8 elements.
- mmooot3euc.inp
-
Uniaxial compression with temperature dependence, C3D8 elements.
- mmoooo3vlp.inp
-
Linear perturbation steps containing LOAD CASE, uniaxial compression, C3D8 elements.
- mctc_trxs.inp
-
Triaxial extension with tension cutoff, CAX4 elements.
- mctc_ucut.inp
-
Tension cutoff, uniaxial compression followed by uniaxial tension, C3D8R and CAX4R elements.
- mctc_psss.inp
-
Tension cutoff, plane strain compression/tension and simple shear, CPE4R elements.
- mctc_btbc.inp
-
Tension cutoff, biaxial tension followed by biaxial compression, C3D8R element.
- mctc_ptpc.inp
-
Tension cutoff, hydrostatic tension followed by hydrostatic compression, C3D8R element.
Abaqus/Explicit input files
Material 1:- mmoooo3jht_xpl.inp
-
Hydrostatic tension, C3D8 elements.
- mmoooo3ltr_xpl.inp
-
Triaxial stress, CPE4R elements (inhomogeneous).
- mmoooo3dte_xpl.inp
-
Triaxial extension, CAX4R elements.
- mmoooo3hut_xpl.inp
-
Uniaxial tension, C3D8 elements.
- mmoooo3gsh_xpl.inp
-
Shear, C3D8 elements.
- mmoooo3ctc_xpl.inp
-
Triaxial compression, CAX4R elements.
- mmoooo3euc_xpl.inp
-
Uniaxial compression, C3D8 elements.
- mmooot3euc_xpl.inp
-
Uniaxial compression with temperature dependence, C3D8 elements.
- mctc_trxs_xpl.inp
-
Triaxial extension with tension cutoff, CAX4R elements.
- mctc_ucut_xpl.inp
-
Tension cutoff, uniaxial compression followed by uniaxial tension, C3D8R and CAX4R elements.
- mctc_psss_xpl.inp
-
Tension cutoff, plane strain compression/tension and simple shear, CPE4R elements.
Transferring results from Abaqus/Standard to Abaqus/Explicit
Material 1:- mmoooo3jht_sx_s.inp
-
Abaqus/Standard analysis, hydrostatic tension, C3D8 elements.
- mmoooo3jht_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mmoooo3ltr_sx_s.inp
-
Abaqus/Standard analysis, triaxial stress, CPE4R elements.
- mmoooo3ltr_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mmoooo3dte_sx_s.inp
-
Abaqus/Standard analysis, triaxial extension, CAX4R elements.
- mmoooo3dte_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mmoooo3hut_sx_s.inp
-
Abaqus/Standard analysis, uniaxial tension, C3D8 elements.
- mmoooo3hut_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mmoooo3gsh_sx_s.inp
-
Abaqus/Standard analysis, shear, C3D8 elements.
- mmoooo3gsh_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mmoooo3ctc_sx_s.inp
-
Abaqus/Standard analysis, triaxial compression, CAX4R elements.
- mmoooo3ctc_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- mmoooo3euc_sx_s.inp
-
Abaqus/Standard analysis, uniaxial compression, C3D8 elements.
- mmoooo3euc_sx_x.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
- sx_s_mctc.inp
-
Abaqus/Standard analysis, uniaxial tension followed by compression, C3D8R element.
- sx_x_mctc_n_y.inp
-
Abaqus/Explicit import analysis, UPDATE=NO, STATE=YES.
Transferring results from Abaqus/Explicit to Abaqus/Standard
Material 1:- mmoooo3dte_xs_x.inp
-
Abaqus/Explicit analysis, triaxial extension, CAX4R elements.
- mmoooo3dte_xs_s.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
- mmoooo3gsh_xs_x.inp
-
Abaqus/Explicit analysis, shear, C3D8 elements.
- mmoooo3gsh_xs_s.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
- mmoooo3ctc_xs_x.inp
-
Abaqus/Explicit analysis, triaxial compression, CAX4R elements.
- mmoooo3ctc_xs_s.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
- mmoooo3ltr_xs_x.inp
-
Abaqus/Explicit analysis, triaxial stress, CPE4R elements.
- mmoooo3ltr_xs_s.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
- mmoooo3jht_xs_x.inp
-
Abaqus/Explicit analysis, hydrostatic tension, C3D8 elements.
- mmoooo3jht_xs_s.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
- mmoooo3hut_xs_x.inp
-
Abaqus/Explicit analysis, uniaxial tension, C3D8 elements.
- mmoooo3hut_xs_s.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
- xs_x_mctc.inp
-
Abaqus/Explicit analysis, uniaxial tension, C3D8R element.
- xs_s_mctc_n_y.inp
-
Abaqus/Standard import analysis, UPDATE=NO, STATE=YES.
![]()
Cast iron plasticity
Elements tested
C3D8
CAX4
CAX4T
CPE4
T3D2
Problem description
Material:
- Elasticity
-
Young's modulus, E = 14.773E6
Poisson's ratio, = 0.2273
- Plasticity
-
Plastic “Poisson's ratio,” = 0.039
Hardening curves: The hardening curves in tension and compression are illustrated in Figure 1.
- Thermal properties
-
Specific heat, = 47.52
Density, = 439.92
Conductivity, k = 9.4
Coefficient of expansion, = 11.0E−6
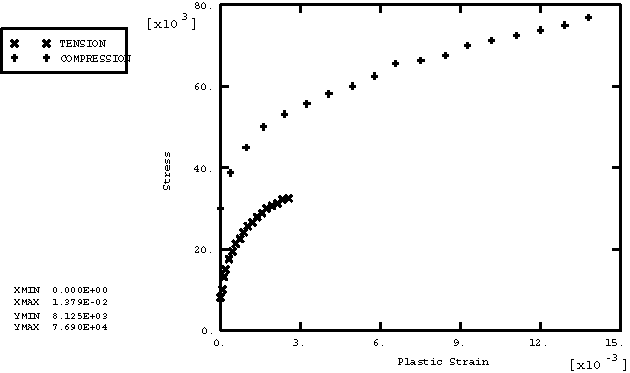
(The units are not important.)
Results and discussion
Most tests in this section are set up as cases of the homogeneous deformation of a single element of unit dimensions. Consequently, the results are identical for all integration points within the element.
Input files
Abaqus/Standard input files
- mciooo3jht.inp
-
Hydrostatic tension, C3D8 elements.
- mciooo3gsh.inp
-
Shear, C3D8 elements.
- mciooo3hut.inp
-
Uniaxial tension, CAX4 elements.
- mcioot3euc.inp
-
Uniaxial compression with temperature dependence, C3D8 elements.
- mctoot3hut.inp
-
Uniaxial tension, coupled temperature-displacement, CAX4T elements.
- mciooo3xmx.inp
-
Inhomogeneous deformation, CPE4 elements.
- mciooo1hut.inp
-
Uniaxial tension and linear perturbation steps containing LOAD CASE, T3D2 elements.
Abaqus/Explicit input files
- mciooo3jht_xpl.inp
-
Hydrostatic tension, C3D8 elements.
- mciooo3gsh_xpl.inp
-
Shear, C3D8 elements.
- mciooo3hut_xpl.inp
-
Uniaxial tension, CAX4R elements.
- mcioot3euc_xpl.inp
-
Uniaxial compression with temperature dependence, C3D8 elements.
- mciooo3xmx_xpl.inp
-
Inhomogeneous deformation, CPE4 elements.
- mciooo1hut_xpl.inp
-
Uniaxial tension, T3D2 elements.
Transferring results from Abaqus/Standard to Abaqus/Explicit
- mciooo3gsh_sx_s.inp
-
Abaqus/Standard analysis, shear, C3D8 elements.
- mciooo3gsh_sx_x.inp
-
Abaqus/Explicit import analysis from mciooo3gsh_sx_s.inp.
- mciooo3gsh_xs_s.inp
-
Abaqus/Standard import analysis from mciooo3gsh_sx_x.inp.
- mciooo3gsh_xx_x2.inp
-
Abaqus/Explicit import analysis from mciooo3gsh_sx_x.inp.
![]()
Soft rock plasticity with porous elasticity
Elements tested
C3D8R, CPE4R
Problem description
Material 1
- Power law–based porous elasticity
-
= 75000.0 = 1.0 = 1.0 = 0. = 0.2 = 0.2 = 1.0 - Plasticity
-
= 60.0 = 50.0 = 1.6 = 0.001 = 0.0007 = 0.25 = 0.05 Tabulated curves are used for defining the compressive and tensile hardening.
(The units are not important.)
Material 2
- Power law–based porous elasticity
-
= 75000.0 = 1.0 = 1.0 = 0. = 0.2 = 0.2 = 1.0 - Plasticity
-
= 45.0 = 45.0 = 1. = 0.001 = 0.0007 = 0.0 = 0.0 Tabulated curves are used for defining the compressive and tensile hardening.
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
- softrockporelatests.inp
-
Hydrostatic compression, uniaxial compression, and shear using materials 1 and 2.
![]()
Soft rock plasticity with linear elasticity
Elements tested
C3D8R, CPE4R
Problem description
Material 1
- Linear elasticity
-
Young's modulus, E = 75.E3
Poisson's ratio, = 0.2
- Plasticity
-
= 60.0 = 50.0 = 1.6 = 0.001 = 0.0007 = 0.25 = 0.05 Tabulated curves are used for defining the compressive and tensile hardening.
(The units are not important.)
Material 2
- Linear elasticity
-
Young's modulus, E = 75.E3
Poisson's ratio, = 0.2
- Plasticity
-
= 45.0 = 45.0 = 1. = 0.001 = 0.0007 = 0.0 = 0.0 Tabulated curves are used for defining the compressive and tensile hardening.
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
Abaqus/Standard input files
- softrocklineelatests.inp
-
Hydrostatic compression, uniaxial compression, and shear using materials 1 and 2.
Abaqus/Explicit input files
- softrocklineelatests_xpl.inp
-
Hydrostatic compression, uniaxial compression, and shear using materials 1 and 2.
![]()
Plasticity model for superelastic materials
Elements tested
C3D8, CPS4, CPS4R
Problem description
Material
- Elasticity
-
= 40000.0 = 0.33 = 32000.0 = 0.33 = 0.041 = 440.0 = 540.0 = 250.0 = 140.0 = 440.0 = 22.0 = 6.7 = 6.7 - Plasticity
-
Tabulated curves are used for defining the yield stress as a function of total strain.
(The units are not important.)
Results and discussion
The results agree well with exact analytical or approximate solutions.
Input files
Abaqus/Standard input files
- superelas_std.inp
-
Tension test.
Abaqus/Explicit input files
- superelas_xpl.inp
-
Tension test.