Double cantilever subjected to multiple base motions

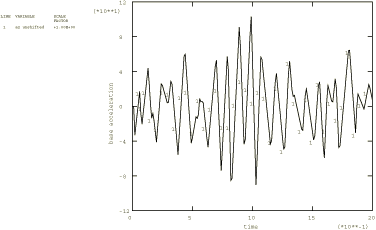
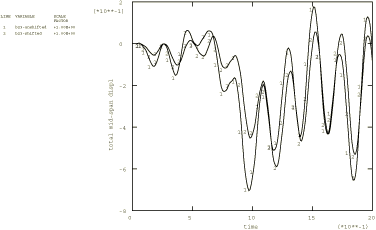
The base acceleration record is shown in Figure 2. Figure 3 shows the (total) displacement response of the midspan node to the unshifted and shifted base excitations predicted using the dynamic procedure.

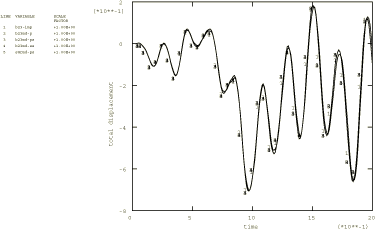
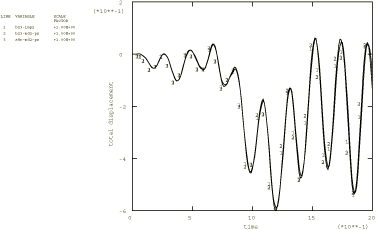
The modal dynamic analysis results agree closely with the implicit dynamic solution. Figure 4 shows the total displacement response of the midspan obtained with beam models using different dynamic procedures, as well as various base motions for modal dynamic analyses, for the case with no time shift. Figure 5 shows the same responses for the case where the motion at the left-end support is delayed by 0.25 second. As a verification exercise the modal dynamic analysis that invokes multiple base motions is repeated using a shell mesh with 10 S8R elements and gives the same results as obtained by the beam model. These solutions are obtained based on superposition of the first six nonzero eigenmodes of the structure. For models with secondary bases the additional low-frequency modes resulting from the unconstrained degrees of freedom at the bases must be taken into account. Abaqus automatically increases the number of eigenfrequencies to keep the number of relevant frequencies constant. However, the eigenmode range used for the modal damping must be extended by the user. The boundary conditions that make up the primary base normally suppress all rigid body motion. If they do not, as occurs in the third model where the primary base is absent, a suitable (negative) shift point must be used in the frequency procedure to avoid numerical problems.

In modal dynamics the default output gives motion relative to the primary base. The sum of this relative motion and the base motion of the primary base yields the total motion. In the absence of primary base motions the relative and total motions are identical. The plots shown in Figure 4 and Figure 5 have been requested appropriately to give total values in all cases.